Mechanical behavior of toilet paper perforation

Costa Vieira, J., Vieira, A. C., Mendes, A. O., Carta, A. M., Fiadeiro, P. T., and Costa, A. P. (2021). "Mechanical behavior of toilet paper perforation," BioResources 16(3), 4846-4861.

Abstract

Perforation is used in multilayer tissue products, such as toilet and kitchen papers, as part of the converting process. Perforation facilitates the detachment of consecutive sheets by the user. The compromise between the strength required to detach a perforated sheet and the strength required to break a sheet affects the perforation efficiency. In this work, the mechanical behaviors of 15 commercial papers from different European producers were studied. A morphological analysis of the materials was performed, followed by the determination of their perforation efficiency (through tensile tests). A qualitative analysis of the cuts quality, along with a quantitative analysis of the same cuts dimensions was performed through an optical system. Finally, the stress concentration in the holes and the influence of the cuts distance were analyzed using a finite element model implemented in Abaqus/Standard finite element software. The results showed that a cut distance of 2.0 mm should not be used in these types of papers, and the perforation efficiency increased with the cut distance, regardless of the number of plies in the toilet paper. The stress concentration factor was also determined to have a limit value of 0.11. Papers above this limit value tear at the perforation line, as desired.

Download PDF

Full Article

Mechanical Behavior of Toilet Paper Perforation

Joana C. Vieira,^a,* André C. Vieira,^b António de O. Mendes,^a Ana M. Carta,^cPaulo T. Fiadeiro,^a and Ana P. Costa ^a

Keywords: Tissue paper; Perforation; Finite element methods (FEM); Mechanical behavior

Contact information: a: Fiber Materials and Environmental Technologies (FibEnTech-UBI), Universidade da Beira Interior, R. Marquês D’Ávila e Bolama, 6201-001 Covilhã, Portugal; b: Center for Mechanical and Aerospace Science and Technologies (C-MAST-UBI), Universidade da Beira Interior, R. Marquês D’Ávila e Bolama, 6201-001 Covilhã, Portugal; c: RAIZ – Instituto de Investigação da Floresta e Papel, Aveiro, 3801-501, Portugal; *Corresponding author: joana.costa.vieira@ubi.pt

GRAPHICAL ABSTRACT

INTRODUCTION

Prior to the invention of toilet paper, people across the world had different methods for personal hygiene. The first record of toilet paper took place in China around 851 AD. Thereafter, no document was found on the use of toilet paper until the 14^th century. During the Ming Dynasty (1368 AD to 1644 AD), special sheets of toilet paper were made for the Imperial court. These toilet paper sheets were made of soft fabric that was cut into 2 ft by 3 ft squares (Bennett 2009).

Joseph C. Gayetty invented the first packaged toilet paper in the United States in 1857. “Gayetty Medicated Paper” was sold in flat-leaf packages, and it contained a hemorrhoid medicine and watermark with its name (Hendrickson 2000). In 1871 Seth Wheeler became the official inventor of toilet roll as it is known today. From this invention, Wheeler held the first patent of the perforated toilet paper roll (Wheeler 1894).

Converting technology produces consumable, finished products from large paper rolls. Converting equipment can perform lamination, printing, embossing, perforation, and packaging operations to produce multi-layer bathroom papers and towels, table napkins, and other disposable products (de Assis et al. 2018).

When a multilayer or single layer paper product such as toilet paper is produced, a perforation process is generally used. Multilayer or single layer tissue papers should be perforated to allow easy dispensing of the paper sheets (pieces of laminated paper between two consecutive perforations) so that a consumer can use it incrementally. This process allows a consumer to detach and use one or more sheets from a toilet paper roll (Schulz and Gracyalny 1998; Paulapuro 2000).

Perforations are formed using a roll with several diagonally arranged blades to perforate the paper sheet. This process occurs at a high speed in the converting machine. Within the perforation line there exist bonding areas, i.e. the uncut area of the perforation process. As the blade is pressed against the paper sheet, it cuts any point of contact. The slots in the blade prevent certain areas of the paper sheet from being cut. The uncut areas of the paper prevent the sheet from tearing prematurely (Baggot et al. 2006). Figure 1 shows a diagram of a perforation blade creating a perforated tissue paper sheet.

Fig. 1. The tissue paper perforation scheme

The tensile strength of perforation is the strength that is required to ensure that the paper separates through its perforation zone. The tensile strength of the perforation should be optimized to allow high-speed production of the multi-layer tissue paper product and to allow the consumer to easily separate individual sheets. In a multilayer tissue paper, the perforation must be done in all layers at the same time to facilitate the detachment of paper sheets by the consumer (Schulz and Gracyalny 1998).

In converting operations, the machine is required to perforate the tissue paper under consistent conditions. The machine should run without vibration or equipment failure and at high processing speeds to reduce the maintenance requirements. In addition, a system capable of changing the adhesion patterns, the sheet length, and the weight in a short time is desirable (Baggot et al. 2006).

The perforation zones must be strong enough to hold together under a certain tension when the consumer wishes to use more than one sheet. The perforation zone must also be weak enough to enable detachment from the roll easily and in a straight horizontal line. This balance is measured using the perforation efficiency. A higher perforation efficiency allows for easier separation of the sheets. The perforation efficiency is determined according to the ISO standard 12625-12 (2010), as seen in Eq. 1,

(1)

where E_pis the perforation efficiency (%), is the average tensile strength of the perforated papers (N/m), and is the average tensile strength of the unperforated papers (N/m).

A hole will affect the stress field near the geometrical discontinuity, and the maximum stress depends on the hole geometry. Therefore, the stress concentration factor geometry due to the perforation geometry will affect the final efficiency. The stress concentration factor is defined as the ratio between the highest value at a geometrical discontinuity and the nominal stress at the minimum cross-section (Carvill 2015).

In this work, several commercial toilet papers with approximately the same blank distance (theoretically 1.0 mm) and different cut distances were tested. The perforation design was studied in terms of its final efficiency. To the authors knowledge, there have been no other studies on this subject.

EXPERIMENTAL

Materials

Fifteen toilet papers with different cut distances were selected. Six of the toilet papers were 2-ply, four of the toilet papers were 3-ply, four of the toilet papers were 4-ply paper, and one of the toilet papers was 5-ply. These toilet papers were identified according to the following legend: XP_i, where X is the commercial toilet paper brand, P_i is the number of plies, and XP_iC_j, where C_j is the cut distance (mm) of the perforation. The values of i = 2, 3, 4, and 5 represent the number of plies, and j = 2, 3, 4, and 5 mm represent the cut distances.

It was previously verified that three of the 2-ply papers tear at other locations than the perforation when they were loaded manually (toilet papers C, D, and E). All the other papers tear at the perforation when they were loaded manually.

Methods

All the toilet tissue samples, and all performed tests were equilibrated in a conditioned room according to ISO 187 (1990) (temperature of 23 ± 1 °C and relative humidity of 50 ± 2%). The grammage of the toilet papers, defined as the mass per unit paper area, was determined and expressed in g/m². The grammage was determined by weighing the paper sample of a known area in agreement according to the ISO standard 12625-6 (2005) using a Mettler Toledo PB303 Delta range analytical balance (Mettler Toledo, Columbus, OH, USA). The thickness was also determined using a FRANK-PTI micrometer for tissue paper (FRANK-PTI GMBH, Birkenau, Germany), where a sheet of paper or a stack of sheets of paper was compressed at a given pressure between two parallel plates according to the ISO standard 12625-3 (2014). The bulk, which is the inverse of the density, was determined by using the grammage and thickness according to the ISO standard 12625-3 (2014).

The morphology of all the commercial toilet papers was evaluated using the MorFi Fiber and Shive Analyzer from Techpap SAS (Gières, France). The morphology analysis provided the fiber length, the fiber width, the fiber distribution, the fiber coarseness, and the fines percentage for the toilet paper samples.

The tensile tests were performed with a Thwing-Albert Vantage^NX universal testing machine (West Berlin, NJ, USA), according to the ISO standard 12625-12 (2010). Each sample was prepared with the perforation in the center. The un-perforated area of the other samples were also prepared and tested (Fig. 2). Both type of samples had a width of 50 mm and a length of 150 mm, to allow a gauge length of 100 mm to carry out the tensile tests and a rate of elongation of 50 mm/min, according to above referred standard.

Fig. 2. The experimental set-up to test the non-perforated and perforated toilet papers

For the distance measurements of the papers, a customized optical system (Mendes et al. 2013, 2014, and 2015) was used. This system consisted in the image acquisition of the surface of the studied papers, with specific conditions of illumination and magnification. After it was properly configured for the application in hand, the optical system allowed for the observation of the elements to be measured using processing tools for this task. In this work, the elements in study (cut and blank distances) were carried out considering 10 different measurements, which were used for the calculation of the corresponding average and standard deviation for all the studied papers.

Numerical Model

In this work, the influence of the cut distance was studied using mechanical simulation tools. The aim was to evaluate how the increased cut distance affected the mechanical strength and the stress concentration around the cuts.

A finite element model was implemented in Abaqus/Standard finite element software (Johnston, RI, USA), using the linear elastic constitutive model to simulate the tensile test on samples with 2, 3, 4, and 5 cut distances. Two Young’s moduli were used, 1.38 and 0.95 MPa, which represented the mechanical properties of the papers with different behaviors AP₂ (tear at the perforation) and CP₂ (tear at other location than perforation). The Young’s modulus values were determined from the respective tensile tests without perforation, as the slope between two specific points in the initial linear part of the load-elongation curve. The coefficient of Poison was estimated to the value of 0.3. The sample geometry was 50.0 mm in width, 100.0 mm in length, and 0.3 mm in thickness. An axial load was applied by controlling a uniform displacement of 10.0 mm of the top surface. The lower surface was constrained to move and rotate in all directions. The CPS4R elements used in these models were 7266, 6085, 5437, and 5194 for the cut distances of 2, 3, 4, and 5 mm, respectively. The geometry of the cuts was an ellipse with a smaller diameter of 0.01 mm and the longer diameter corresponded to each cut distance.

RESULTS AND DISCUSSION

The commercial toilet papers samples were first subjected to a physical and morphological characterization. Table 1 shows the grammage, thickness, and bulk results for all the toilet papers, which were determined according to the previously mentioned standards.

The grammage ranged from 32.4 g/m² to 76.0 g/m². These values corresponded to a composition of the number of plies. The thickness and bulk values varied by 58% and 70%, respectively, due to the embossing type and the number of plies. Also, as can be seen in Table 1, an increase in the number of plies did not imply an increase in thickness and / or bulk. The highest bulk was found for the toilet paper (FP₂) with the low number of plies, the smallest grammage value, and the major thickness. On the other hand, the smallest bulk was found for a 4-ply toilet paper (MP₄), with the second largest grammage, and one of the thicknesses with the lowest value. This analysis reinforces the impact that the embossing operation has in the z-direction. Most of the lowest bulk values were found for the toilet papers with a higher number of plies, and this reveals that the final structure of the toilet paper is more compact, with a small expansion in the z-direction. Hermans et al. (2009) explain how the empty space between the different sheets on a toilet paper can be maximized by the way they are combined. The combination of the plies with deco and the micro embossing patterns, is where the greatest void volume is achieved, because between layers with the same embossing pattern, the voids are mainly due to the creping of each ply. The points of contact between them prevent the structure from collapsing when compressed or wet. This is in line with the results obtained.

The morphological characterization and the fiber distribution, by length weighted in length and width, of the commercial toilet papers samples are presented in Table 2 and Fig. 3. Analyzing the different morphological characteristics revealed that all the toilet paper samples were composed mainly of short, hardwood fibers. These results are in agreement with those found in the literature (Niskanen 1998). As a complement to the information obtained in Table 2, Fig. 3 illustrates the percentages of the short and long fibers in the toilet paper samples. As expected, this small percentage of long fiber is used to improve the paper strength and machine runnability during the tissue paper production process.

The toilet paper CP₂ stood out from the others for its higher average value of width (23.2 m) and coarseness (9.98 mg/100 m) of fibers. In addition, the CP₂ sample also had a notable width distribution, particularly above 30 m (Fig. 3). The CP₂ sample was not the sample with the highest length distribution (>2000 m); it can be inferred that the CP₂ paper was composed of some recycled fiber, which contributed to the sample’s low Young’s modulus value (0.95 MPa).

Fig. 3. The fiber distribution by a) length weighted in length (mm) and b) width (m)

As shown in Table 3, the MP₄ paper had the highest perforation efficiency and the longest cut distance, at 79.4% and 4.92 mm, respectively. The DP₂paper had the lowest perforation efficiency and the shortest cut distance, at 46.4% and 1.48 mm, respectively. In general, for papers with the same number of plies, a greater cut distance yielded a higher perforation efficiency. Of the studied papers, only the CP₂, DP₂, and EP₂ papers tore at other locations than the perforation, as mentioned above. These papers had the lowest cut distance, as did the AP₂ paper, which did not tear at the perforation. Although the AP₂ and CP₂ papers had the same cut distance (2.31 mm), they had different Young’s modulus values of 1.38 MPa and 0.95 MPa, respectively. The CP₂paper had the lowest Young’s modulus value.

Figure 4 shows the representative images of the four cut distances that were obtained from the studied toilet papers.

Fig. 4. Images of the a) C₅, b) C₄, c) C₃, and d) C₂ cut distances that were obtained with the customized optical system

During the perforation process, the blade passes through the paper sheet. The perforating blade becomes dull during the process because of its physical contact with the paper. This will cause incorrectly perforated or absent holes, so that when the user separates a sheet from the toilet paper roll, tearing is likely to go off the designated pattern of perforation, as illustrated in Fig. 5. The cellulose fibers are not cut but mashed and piled up at the bottom of the perforated hole. This layer of mashed fibers closes the back of the holes between the two services of the toilet paper. If the perforation is done with too much pressure, it can damage the bonding areas. These experiments are in line with the previous results of Gattuso (1989).

Fig. 5. Image of an incorrect perforation

The cutting efficiency (measured cutting distance) was compared to the target cutting distance if it was made with a perfect blade (without abrasion), as shown in Fig. 6.

As shown in Fig. 6, the cuts were effective despite some variability for the 2-ply samples. This variability can be justified by the low mechanical resistance of its structure, because of the reduced number of plies.

Fig. 6. Effectiveness of the cutting

Another important factor to understand is the perforation efficiency evolution with the measured cut fraction, as shown in Fig. 7.

Fig. 7. Perforation efficiency against the measured cut fraction of the toilet papers

Figure 7 shows the impact that the cutting distance had on the perforation efficiency. Overall, the samples with a larger cutting distance had a greater perforation efficiency. As mentioned before, the 2-ply papers negatively influenced this correlation because the cutting distance was the smallest and some toilet papers had a tear at other location than perforation.

When holes were present, they effectively reduced the cross-sectional area. Instead of the full width, the resistant width was smaller. The nominal stress (N/m), which considered the load per unit width, is the ratio between the average maximum force of non-perforated papers and the resistant length, as seen in Eq. 2,

(2)

where is the nominal stress (N/m), is the average maximum force of non-perforated papers (N), L is the resistant width (mm) of the tensile test samples, CD is the cut distance (mm), and NH is the average number of holes in the same test sample. Since the tensile strength of non-perforated paper is higher than that of perforated paper due to a smaller resistant width, it follows that the paper will tear along cross sections where holes are present. While this correctly calculates the average stress, it assumes that the stress between holes is uniform (and equal to the nominal stress). However, the stress field between holes is not uniform. It is well known that a hole will lead to a heterogeneous stress field around the geometrical discontinuity and this depends on the geometry of the hole (i.e. the ratio between the width and the hole diameter). Therefore, the geometry of the cut will influence the maximum stress near the hole. Stress concentrations describe the stress state at abrupt changes in geometry, where the stress field is non-uniform. Figure 8 shows the effect of the stress concentration where, analogous to lines of pressure in a fluid flow around an immersed body, lines of force (or “flow”) around the holes become concentrated, where the absence of material is unable to transmit force.

Fig. 8. Effect of stress concentration

A stress concentration factor (k) is applied to the nominal stress to calculate the maximum stress as seen in Eq. 3,

(3)

When the stress at any location in the paper exceeds the paper’s strength a tear is initiated and follows the line of highest stress, as seen in Eq. 4,

(4)

The resistant width is given by Eq. 5,

(5)

where BD is the blank distance, which in this case is equal to 1.0 mm, and the stress concentration factor is given by Eq. 6:

(6)

In Fig. 9, the stress concentration factor, calculated according to Eq. 5 based on the results presented in Table 3, is presented as function of the cut distance. The perforated papers that had a stress concentration above 0.11 were prone to tear at other location than perforation.

Fig. 9. Stress concentration factor (k) vs. the cut distance (mm)

The red line in Fig. 9 illustrates the stress concentration limit of 0.11. The AP₂ toilet paper is the limiting case in this set of samples, as it is on the red line. The papers below the red line tear at the perforation, as they were supposed to.

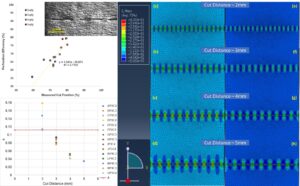
More accurate solutions can be obtained by Finite Element Methods (FEM), where analytic solutions are not possible with complex geometries. Considering the elliptic dimensions mentioned in the Numerical Model section, the software calculates the von-Mises stress field.

In Fig. 10, the colored scale shows the regions in red that correspond to higher equivalent von-Mises stress. The blue regions are the lower equivalent von-Mises stresses, equal to the nominal stresses present in non-perforated stresses. As the cut distance increased, the region of stress heterogeneity became wider due to a sharper geometry. Hence, the transition from higher stresses to lower stresses is cushioned. In Figs. 10a, 10b, 10c, and 10d, the material was less rigid. This transition from higher stresses to lower stresses was also more softened when compared to a stiffer material (Figs. 10e, 10f, 10g, and 10h). Furthermore, for higher cut distances for the regions of higher stress (in red) were visible, which indicates that this paper will preferably cut on this perforated region.

Fig. 10. The von-Mises Stress fields where the tear at perforation of the AP₂paper is represented by a), b), c), and d) at the cut distances of 2, 3, 4, and 5, respectively. The tear at perforation of the CP₂ paper is represented by e), f), g), and h) at the cut distances of 2, 3, 4, and 5, respectively.

CONCLUSIONS

In this work, 15 commercial toilet papers from different European suppliers with various numbers of plies and cut distance were selected. The morphological analysis of the papers revealed that they present several fibrous compositions that resulted in different mechanical responses.
An optical method was used to measure the cut distance, the blank distance, and the quality of the perforation for the toilet paper samples.
In general, the cut distance had a great influence on the result of the perforation efficiency. The sample with the highest cut distance (4.92 mm) also had the greatest perforation efficiency (79.4%). It was also determined that a cut distance of 2.0 mm should not be used in this type of paper, in order to minimize the tearing of the paper away from the perforation.
The toilet paper samples with a stress concentration factor above 0.11 experienced tear at other locations than at the perforation. The toilet paper samples with a stress concentration factor below 0.11 tore at the perforation.
The FEM analysis revealed that the region of stress heterogeneity became wider due to a sharper geometry, as the cut distance increased. Moreover, regions of higher stress were visible for the upper cut distance, which indicated that the paper will preferably cut on this perforated region.

ACKNOWLEDGMENTS

The authors gratefully acknowledge the funding of this work that was carried out under the Project InPaCTus – Innovative products and technologies from eucalyptus, Project No. 21874 funded by Portugal 2020 through European Regional Development Fund (ERDF) in the frame of COMPETE 2020 nº246/AXIS II/2017.

The authors also acknowledge UID Fiber Materials and Environmental Technologies (FibEnTech-UBI) on extent of the project UIDB/00195/2020, funded by the Fundação para a Ciência e a Tecnologia (FCT).

REFERENCES CITED

Baggot, J. L., Gropp, R. F., and Wojcik, S. (2006). “System and method for severing or perforating a web,” U. S. Patent No. 20060014616.

Bennett, H. (2009). “Ever wondered about the history of toilet paper?” Washington Post (https://www.washingtonpost.com/wp-dyn/content/article/2009/05/31/AR2009053102217_2.html), Accessed 14/01/2019.

Carvill, J. (2015). Mechanical Engineer’s Data Handbook, Butterworth Heinemann, Oxford, United Kingdom.

de Assis, T., Reisinger, L. W., Pal, L., Pawlak, J., Jameel, H., and Gonzalez, R. (2018). “Understanding the effect of machine technology and cellulosic fibers on tissue properties – A review,” BioResources 13(2), 4593-4629. DOI: 10.15376/biores.13.2.DeAssis

Gattuso, C. F. (1989). “Laser perforation for computer paper,” Rochester Institute of Technology, Rochester, NY, USA.

Hendrickson, R. (2000). The Facts on File dictionary of American Regionalisms, Facts on File, New York, NY.

Hermans, A., Perkins, J., Ko, Y., Kainth, A., Boudrie, L., Baggot, J., and Smith, M. (2009). “Multiple ply tissue products having enhanced interply liquid capacity,” Neenah, WI, U. S. Patent No. 7,524,399 B2.

ISO 12625-3 (2014). “Tissue paper and tissue products – Part 3: Determination of thickness, bulking thickness and apparent bulk density and bulk,” International Organization for Standardization, Geneva, Switzerland.

ISO 12625-6 (2005). “Tissue paper and tissue products – Part 6: Determination of grammage,” International Organization for Standardization, Geneva, Switzerland.

ISO 12625-12 (2010). “Tissue paper and tissue products – Part 12: Determination of tensile strength of perforated lines – Calculation of perforation efficiency,” International Organization for Standardization, Geneva, Switzerland.

ISO 187 (1990). “Paper, board and pulps — Standard atmosphere for conditioning and testing and procedure for monitoring the atmosphere and conditioning of samples.” International Organization for Standardization, Geneva, Switzerland.

Mendes, A. O., A., Fiadeiro, P. T., Costa, A. P., Amaral, M. E., and Belgacem, M. N. (2013). “Retro-diffusion and transmission of laser radiation to characterize the paper fiber distribution and mass density,” in: Proceedings Volume 8785: 8th Ibero American Optics Meeting and 11^th Latin American Meeting on Optics, Lasers, and Applications, Porto, Portugal.

Mendes, A. O., A., Fiadeiro, P. T., Costa, A. P., Amaral, M. E., and Belgacem, M. N. (2014). “Study of repeatability of an optical laser system for characterization of the paper fiber distribution and mass density,” in: Proceedings Volume 9286: Second International Conference on Applications of Optics and Photonics, Aveiro, Portugal.

Mendes, A. O., Fiadeiro, P. T., Costa, A. P., Amaral, M. E., and Belgacem, M. N. (2015). “Laser scanning for assessment of the fiber anisotropy and orientation in the surfaces and bulk of the paper,” Nordic Pulp & Paper Research Journal 30(2), 308-318. DOI: 10.3183/npprj-2015-30-02-p308-318

Niskanen, K. (1998). Papermaking Science and Technology Bookseries: Volume 16- Paper Physics, Fapet Oy, Helsinki, Finland.

Paulapuro, H. (2000). Papermaking Science and Technology Bookseries: Volume 18- Paper and Board Grades, Fapet Oy, Helsinki, Finland.

Schulz, G., and Gracyalny, D. T. (1998). “Method and apparatus for pinch perforating multiply web material,” U. S. Patent No. 5755654.

Wheeler, S. (1894). “Wrapping or toilet paper,” U. S. Patent No. 511983.

Article submitted: December 20, 2020; Peer review completed: March 27, 2021; Revised version received and accepted: March 31, 2021; Published: May 13, 2021.

DOI: 10.15376/biores.16.3.4846-4861